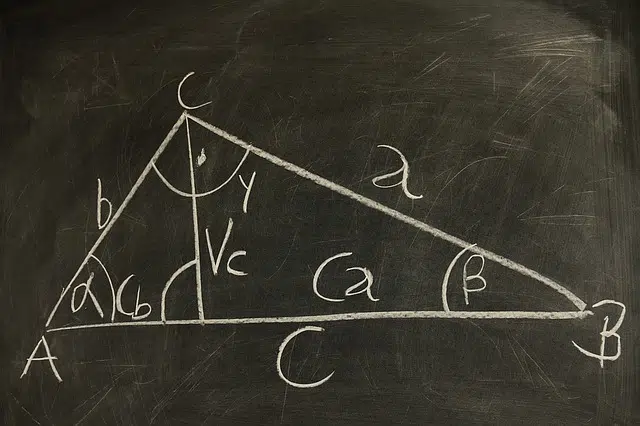
Trigonometry performs calculations of the elements of a triangle.
The first step before entering fully into the analysis of the meaning of the word trigonometry is to proceed to establish its etymological origin. In this sense we have to explain that the aforementioned is found in Greek where we can see how it is formed by the union of trigonon which is equivalent to "triangle", metron which can be defined as "measure" and tria which is synonymous with "three". .
Trigonometry is the subdivision of mathematics that is responsible for calculating the elements of triangles . For this, it is dedicated to studying the relationships between the angles and sides of triangles.
Units used by trigonometry
This specialty intervenes in various areas of mathematics in which it is necessary to work with precision. Trigonometry, however, has a wide variety of applications. It allows, for example, to measure the distances between two locations or celestial bodies using triangulation techniques . Trigonometry is also applied in satellite navigation systems.
There are three units that trigonometry uses to measure angles: the radian (considered the natural unit of angles, establishing that a complete circle can be divided into 2 pi radians), the gradian or centesimal degree (which allows the circumference to be divided into four hundred centesimal degrees) and the sexagesimal degree (used to divide the circumference into three hundred and sixty sexagesimal degrees).
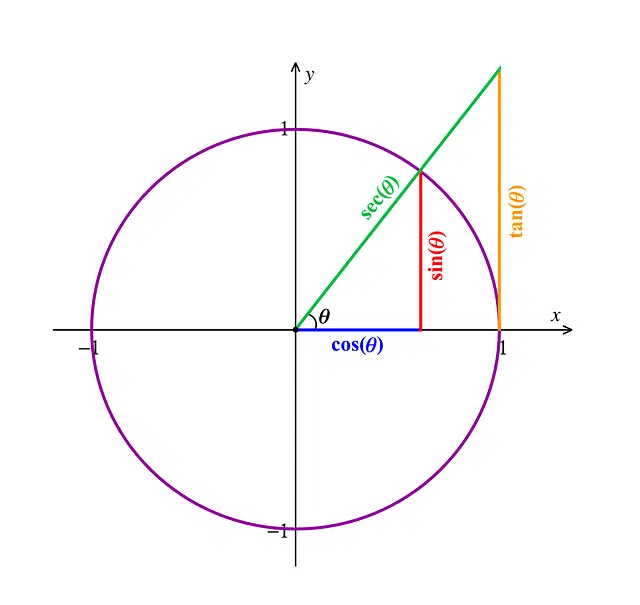
The sine, cosine and tangent are the main trigonometric ratios.
Trigonometric ratios
The main trigonometric ratios are three: the sine (which consists of calculating the ratio between the opposite leg and the hypotenuse), the cosine (another ratio but, in this case, between the adjacent leg and the hypotenuse) and the tangent (the ratio between both legs: the opposite over the adjacent).
The reciprocal trigonometric ratios, on the other hand, are the cosecant (the reciprocal ratio of the sine), the secant (the reciprocal ratio of the cosine), and the cotangent (the reciprocal ratio of the tangent).
Trigonometry and goniometric circumference
These are the different types of main trigonometric ratios, but we cannot ignore that there are also other fundamental elements within this branch of Mathematics that concerns us now. Specifically, we are referring to the trigonometric ratios of any angle.
The latter would lead us to talk about what is known as a goniometric circle, which is characterized by the fact that its radius is the unit itself and its center is none other than the origin of the relevant coordinates. All of this without forgetting either that what the coordinate axes do is delimit four quadrants that are numbered in the opposite direction to that indicated by the hands of a clock.
Classification according to modality
In addition to all the above, we cannot ignore the existence of two types of trigonometry. Thus, first of all, we would have the so-called spherical trigonometry, which is that part of Mathematics that focuses on proceeding to the study of what spherical triangles are.
Secondly, there is also what is known as plane trigonometry. In this case, as its name indicates, it is that science that has the various flat triangles as its object of analysis and study.
Finally, the equality that involves trigonometric functions and that are verifiable for any value of the variables (the angles on which the functions are applied) is known as trigonometric identity.
