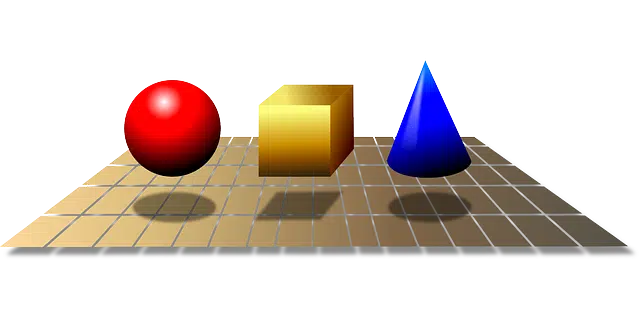
In a three-dimensional object, three coordinates are required to locate a point inside it.
The adjective three-dimensional is used to describe something that has three dimensions . To understand what the notion refers to, therefore, it is necessary to understand the concept of dimension .
In the context of physics and geometry , the idea of dimension refers to the smallest number of coordinates needed to locate a point. A line, in this sense, is one-dimensional : a coordinate is enough to locate any point on it. The plans, on the other hand, have two dimensions since it is essential to know the longitude and latitude for the location of a point.
Characteristics of a three-dimensional object
Continuing with the same reasoning, three-dimensional objects require knowledge of three coordinates to find a point inside them. It is often said that the space that surrounds us is three-dimensional, although there are more dimensions (there are those who include time as another dimension, for example).
Broadly speaking, it can be noted that in a three-dimensional space height (or depth), length and width are present. Knowing these three coordinates, it is possible to locate a point in space .
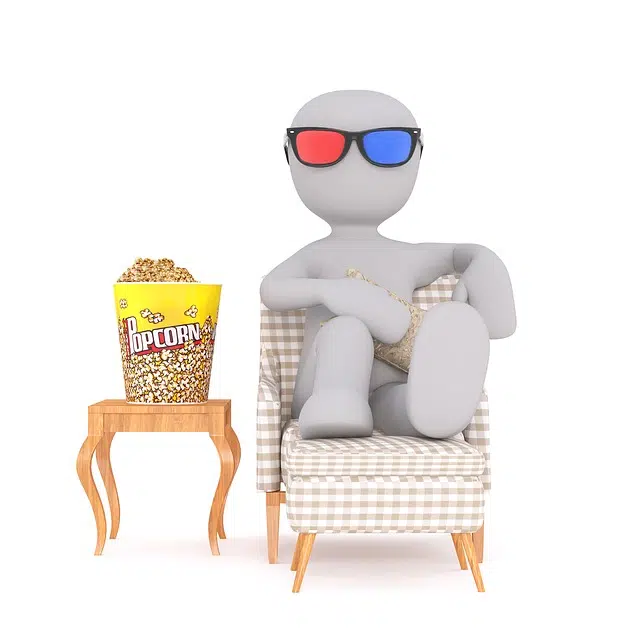
The three-dimensional effect in cinema is achieved through simulation.
A phenomenon produced by simulation
Currently, three-dimensionality usually appears as an effect or phenomenon produced by two-dimensional objects, such as a television screen. In these cases, the three-dimensional is a simulation that is achieved from the projection of certain data. A person wearing corresponding glasses in a movie theater and watching a 3D movie projection can “feel” that the action takes place in a three-dimensional space and not on a screen, since the images appear to be projected in three dimensions.
This type of three-dimensional effect is usually called stereoscopic , since it is achieved by projecting two simultaneous images, one for each eye, each from a slightly different perspective . In other words, although for many 3D is nothing more than a fad among filmmakers and video game developers, it manages to make the content on the screen less abstract, since it represents it in a way that is much closer to what our eyes perceive on the screen. the reality.
Difficulties perceiving the three-dimensional effect
Of course, not everyone can enjoy three-dimensional content: on the one hand there are people who have lost an eye or who have certain health problems that prevent them from correctly perceiving depth ; On the other hand, this effect causes headaches for some, especially after exposures of several hours. These drawbacks make it difficult to spread 3D as a basic form of projection of audiovisual content, but this does not prevent it from gaining more and more strength in the market.
Given the new awakening of stereoscopic 3D (let's not forget that its origins date back more than a century, when in 1833 a British physicist named Charles Wheatstone created the "Mirrorscope" to view images in three dimensions), the public began to have difficulty in understand the meaning of the term "three-dimensional", since until then it was limited almost exclusively to the environment of computer generated graphics, such as those used for Pixar films.
The three dimensions in video games
In the field of video games , three dimensions became the norm in the mid-90s, largely thanks to the success of consoles such as the Nintendo 64, the Playstation and the Dreamcast. However, the experiences of a three-dimensional game are very different from those that a two-dimensional one can offer; For this reason, after "the 3D fever" two-dimensional games appeared again, and currently there is a greater variety on the market.
As a curious fact, nowadays it is normal to use three-dimensional graphics to create two-dimensional content , since it is enough to use an orthogonal projection to cancel the perspective (if the camera does not perceive the deformation of the objects along the Z axis, then the sensation of depth, even though we are viewing a three-dimensional model).
