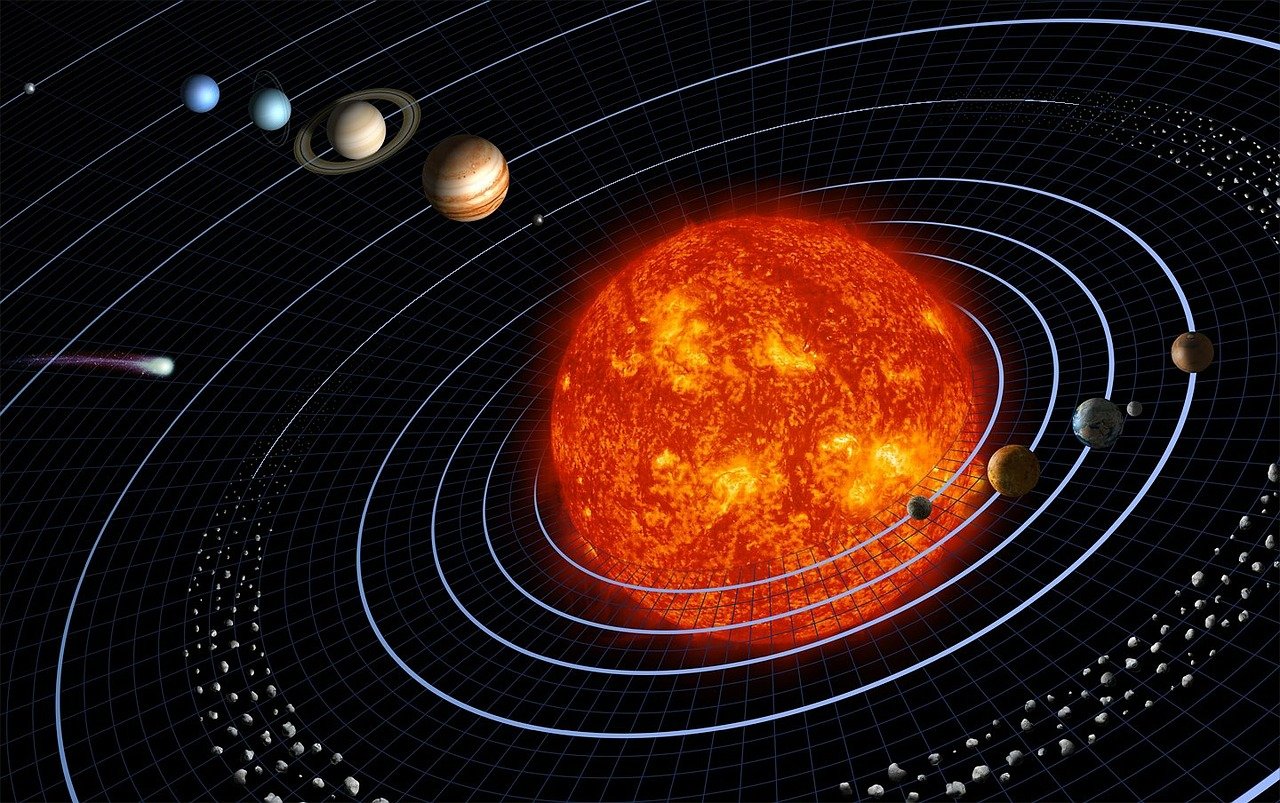
Translation is the movement that the stars make when orbiting.
Translation is a concept with several formal definitions, although its use is usually linked to the movement of translation , which is that developed by bodies that trace curves of wide radius in comparison to their respective dimensions.
For astronomy , the translation of the stars is the movement they develop while they orbit . In this sense, the movement of our planet around the Sun is known as the Earth 's translation, which it performs in an elliptical orbit with a perimeter of about nine hundred and thirty million kilometers.
Various uses of the term translation
The term translation also allows us to name the actions and consequences of changing the location or space of a person or an object. For example: “The company decided to transfer Manuel to the Lima branch,” “The government refuses to transfer the treasure found off the coast of the capital.”
Translation is, on the other hand, the translation into a different language: “I need the translation of this document to be exact and leave no room for erroneous interpretations,” “I have been commissioned to translate a complex scientific text that will take me several weeks of work.” job" .
In the field of grammar , translation is a constructive figure that is specified with the use of a verbal tense beyond its usual meaning. For rhetoric , meanwhile, translation is synonymous with metaphor (the use of a term or phrase about an idea, without denoting it literally but suggesting a comparison for aesthetic purposes or to facilitate its understanding): “The sun, that coin of gold we need to live.”

The idea of translation can be associated with the concept of translation.
The concept in geometry
When talking about moving geometric figures, whether two-dimensional or three-dimensional, in space, it is common for complications to arise when concepts such as rotation or orientation come into play. For example, depending on the configuration you are working with, whether on a computer or in theory, the order in which these operations are applied can affect the result ; In other words, the final position may vary.
To understand the reasons, it is necessary to first deal with the concept of local and universal (or world) coordinates . The first case refers to the orientation of the coordinate axes , which in the case of a three-dimensional space are usually called y , x and z ; This directly affects the perception of the rest of the elements and their location (up, to the right, in front, etc.). The complexity lies in the fact that this orientation can be different for each figure, and in turn all of them can be different from the universal one.
To give a practical example, if a person lies down in the middle of a busy street, what was previously in front of them will become "below", since it will be in front of the soles of their feet , which in their local system coordinate corresponds to its base. On the other hand, each of the subjects present in that scene will have a particular perception of the location of the others, and the world itself will maintain its axes aligned with a different orientation.
Returning to the order of operations , if we asked a person to take four steps forward and when they reached their destination we told them to rotate 90° to the right, they would surely give us a resulting point very different from the one we would obtain by rotating first. and moving afterwards, since their local orientation would be affected before walking, so their "front" would face another direction.
If, on the other hand, we ignored the local orientation of said individual and always relied on the universal one, both results would coincide, but the degree of abstraction would increase, since the steps would be taken towards "the front" with respect to the world, which would remain motionless, since our eyes the person would move to their left.
