
An infinite series is a succession of elements that has no end.
A series is a succession of elements that, when ordered, maintain a certain link between themselves. The notion of infinity , for its part, is linked to that which lacks an end .
An infinite series , therefore, is a string of units that has no end . The opposite concept is that of a finite series , which is characterized by ending at a certain moment.
Examples of infinite series
We can understand the notion of an infinite series if we think about certain numerical series . Let's take the case of the numerical series composed of numbers multiples of 2 . This series is an infinite series since the numbers multiples of 2 are infinite: 0, 2, 4, 6, 8, 10, 12...
Series can be understood as sets . The numerical series of odd positive numbers less than 10 , in this sense, is the set that includes the numbers 1, 3, 5, 7 and 9 . As you can see, this is a finite series. On the other hand, if we wanted to refer to the series of odd numbers , it will be an infinite series : a set with infinite components.
Since numbers are infinite, we can list all kinds of infinite number series. It is even possible to consider infinite descending series: for example, if we mention the series composed of numbers less than 1 : 0, -1, -2, -3, -4, -5, -6...
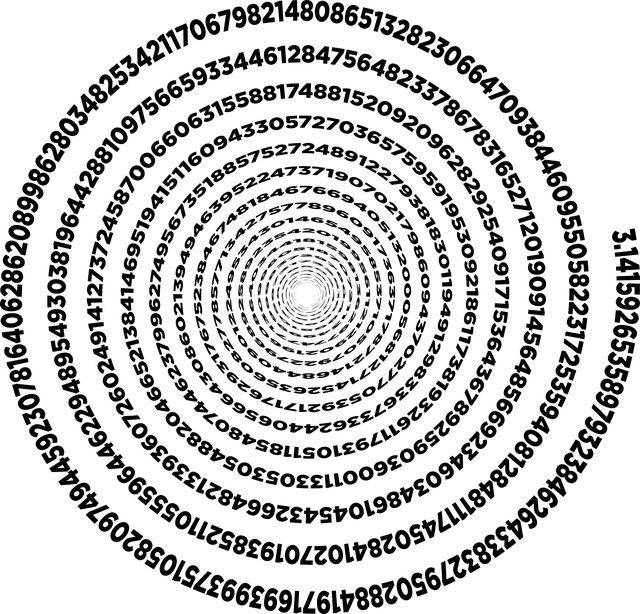
The irrational number Pi is made up of an infinite series of digits.
Some of the most significant
In addition to all of the above, we cannot ignore the fact that there are many and diverse types of infinite series that exist. However, among the most significant we can highlight, for example, the following:
– Harmonic series .
– Geometric series . Under this name there is, for example, an infinite type series that is characterized by the fact that each term is obtained from what is the multiplication of the previous term by a certain constant.
– Convergent series . When it comes to determining whether an infinite series is convergent or not, you can use various tools. Specifically, among the most common are the p-series, which are sums of functions; the geometric series theorem, the direct comparison criterion, the comparison criterion by step of the quotient limit, the Cauchy integral criterion, the d'Alembert criterion and the Leibniz criterion, among many others.
Rules, formulas and algorithms in infinite series
Typically, in the field of mathematics , infinite series arise from different algorithms, formulas or rules. In this way infinite series can be used to represent functions .
One of the most important figures in the field of infinite series was and is the Swiss mathematician and physicist Leonhard Euler (1707 – 1783), who is considered the most important mathematician of the 18th century . In the case at hand, we must highlight the fact that he chose to undertake exhaustive research into the development of calculus and that was what led him to establish the mathematical constant as e, which he proceeded to represent not only as a fraction continuous but also as a real number or an infinite series.
