 The term obtuse comes from the Latin obtūsus , in turn derived from the verb obtundĕre (which can be translated as "dull" or "blunt" ). The notion is used in different ways according to the context.
The term obtuse comes from the Latin obtūsus , in turn derived from the verb obtundĕre (which can be translated as "dull" or "blunt" ). The notion is used in different ways according to the context.
The first meaning of obtuse mentioned by the Royal Spanish Academy ( RAE ) in its dictionary refers to that which is blunt ; That is, it has no point . An obtuse object, in this framework, lacks a pointed end. For example: "The expertise determined that the victim was hit with an obtuse element."
The use of obtuse is more common to refer to the dull or brute individual . The obtuse subject, therefore, has difficulty understanding or reasoning about issues that are simple for the majority . The concept also qualifies someone who is clumsy, limited or stupid : "The owner of the company is an obtuse man who inherited the fortune of his parents," "Don't be obtuse and listen to your coach, who knows what you are dealing with." speaking" , "We are at the mercy of an obtuse government that fails to solve the most urgent economic problems."
In the field of geometry , the idea of obtuse angle appears. This is the name given to the angle that measures more than 90° but less than 180° . The obtuse angle, thus, is greater than the right angle and less than the straight angle.
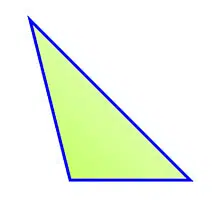
A triangle that has one obtuse angle and two acute angles is called an obtuse triangle or an obtuse angle triangle . Since the interior angles of a triangle always have to add up to 180°, it is not possible for a triangle to have more than one obtuse angle.
The obtuse triangle belongs to the group of so-called oblique or oblique triangles , which are characterized by not having any right angles (90°). This means that it cannot be solved using the Pythagorean theorem as we can do with right triangles; On the contrary, its resolution requires the application of the laws of sines or cosines, depending on the type of triangle.
 Another of the triangles found in this group is the acute one , which is made up of three angles less than 90°, that is, acute , and this gives it its name. In any triangle there is the concept of centroid (or centroid ), which is defined as the intersection of all the hyperplanes that cut the object (in this case, the triangle) into two parts of equal volume .
Another of the triangles found in this group is the acute one , which is made up of three angles less than 90°, that is, acute , and this gives it its name. In any triangle there is the concept of centroid (or centroid ), which is defined as the intersection of all the hyperplanes that cut the object (in this case, the triangle) into two parts of equal volume .
When talking about the centroid of triangles, we must mention the medians (also known as transversals of gravity ), the three segments that go from each of the vertices to the midpoint of its opposite side. In other words, if we cut each side in half , the median is drawn from there to the opposite vertex, that is, the only one that does not touch that side.
There is also the incenter , which is achieved by drawing a circle that touches the three sides of the triangle without leaving it, and then identifying its central point. The orthocenter , on the other hand, is the point at which the lines containing the heights of the triangle intersect. The circumcenter , finally, is also called the circumcircle and is obtained by drawing a circle that passes through all the vertices of a figure and contains it inside.
It is worth mentioning that these last two concepts distinguish the obtuse triangle from the acute one, since in the latter it is observed on the inside, while in the former, on the outside. To calculate the aforementioned heights, we must draw a perpendicular segment from each side towards the opposite vertex; While in an acute triangle the intersection occurs inside, in an obtuse triangle it takes place outside of it, and this explains the previous difference.
