The notion of moment of inertia is used in the field of physics . To understand what the expression refers to, it is first important to understand the terms that make it up.
In the specific field of physics, momentum refers to the quantity of movement : it is the magnitude resulting from the multiplication of the mass of a body by its speed. Inertia , meanwhile, is the property of an object to maintain its state of motion or rest unless a force acts on it.
According to the dictionary of the Royal Spanish Academy ( RAE ), the moment of inertia is the sum of the products obtained by multiplying the mass of the different elements of a body by the square of the distance towards its axis of rotation.
In other words, the moment of inertia is linked to how the mass of the body is distributed with respect to a certain axis of rotation . It is determined, in this way, according to the body geometry and the position of the axis, beyond the forces that produce the movement.
It is important to note that inertia can be understood as the resistance exerted by the body to an alteration of movement. The moment of inertia, in this framework, is analogous to the moment of the inertial mass in a uniform and rectilinear movement.
A body can have different axes of rotation , distributed in different areas of its structure . That is why the same object can have several moments of inertia.
It is important to understand that the axes of rotation that are usually used or taken as a reference in the field of physics are imaginary, although it may be the case that they coincide with material objects that act in this way. When we rotate the different parts of our body, for example, there are no cylindrical bones around which we make these movements, but rather muscular restrictions that guide them.
The axes of rotation are, therefore, imaginary resources that are used in the field of physics to understand these movements and be able to make calculations related to them, both to study each aspect of them and to anticipate or provoke them.
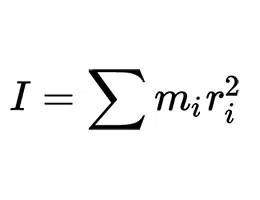Regarding the equation used to express the moment of inertia, we can say that there are several, each with different purposes. If we take an example of the basic definition, stated in a previous paragraph, the mathematical notation of it is found in the image on the right.
Here we find the variables: I , which represents the moment of inertia itself; m , the masses of the particles; r , the minimum distance between the axis and each particle. The symbol Σ , on the other hand, is what is called summation , although it can also be used in feminine, summation. It is a notation used to denote the sum of a succession of terms that are expressly delimited.
Another name by which summation is known is sigma notation , precisely because it uses the eighteenth Greek letter so called. Although it is a sum, we should not use these terms interchangeably, since the sum has well-defined rules. While addition is an operation that can relate any group of numbers, in any quantity, the addition must respect an initial value i (its lower limit) and a final value n (its upper limit>, respecting that i is less than or equal to to n .
The limits should not be expressed in the moment of inertia equation because the sum includes all the elements, that is, the masses of all the particles in the system .