 In the field of mathematics , the expression that refers to a division is called a fraction . The fraction 1/3, for example, implies that the number 1 is divided into 3 (or, put another way, 1 divided by 3). Two or more equivalent elements, meanwhile, are similar or equal .
In the field of mathematics , the expression that refers to a division is called a fraction . The fraction 1/3, for example, implies that the number 1 is divided into 3 (or, put another way, 1 divided by 3). Two or more equivalent elements, meanwhile, are similar or equal .
To construct a mathematical fraction we need to have two components : a numerator and a denominator . In the previous paragraph the example 1/3 is mentioned, which we must read "one third"; In this case we have a numerator of value 1 and a denominator of value 3 . The meaning of such a pair is that we are faced with the third part of an integer, a quantity that to reach the other must be multiplied by three.
It is worth mentioning that the numerators and denominators must always be integers with the exception of zero, that is, elements of the set that has the natural numbers from least infinite to most infinite . Without delving into too technical issues, it is enough to observe the concept of a fraction to understand this rule: given that in itself it expresses a reason, and that the process of dividing its numerator by its denominator often gives us a result with a comma, it would be illogical build it with decimal numbers.
To read a fraction it is necessary to know a special type of word : the numeral . When we write a number we have two options: use the appropriate figures according to the system used or write their names with words, and for this there are numerals.
Numerals are proper names to designate numbers; In other words, they are nouns that are used to refer to them through written or spoken language. There is more than one type of numeral, and the use of one or the other depends on the mathematical concept that we want to express in words. For example, cardinal numerals (also known as common numerals ) are what we use every day to mention numbers when we need to count objects: one, two, three, etc.
In the case of fractions, both equivalent and any other, cardinal numerals are used to refer to their numerator. On the other hand, there are fractional numerals , which are also known as partitive numerals , which serve to express the division of a whole into several parts: half, third, fourth, etc. The denominator of a fraction is read using these terms.
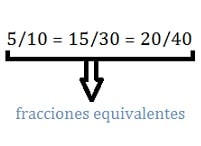
Equivalent fractions , in this way, are those that, although written differently, represent the same quantity . 5/10 , 15/30 and 20/40 , to name a few cases, are equivalent fractions. Let's look at a verification that is obtained by dividing its numerators by its denominators:
5/10 = 0.5
15 / 30 = 0.5
20 / 40 = 0.5
It can be stated that these fractions ( 5/10 , 15/30 and 20/40 ) are equivalent fractions since all three indicate the same quantity: 0.5 .
 A simple way to find out if two or more fractions are equivalent is to multiply the numerator and denominator of each of them by the same number. This process is known as amplification .
A simple way to find out if two or more fractions are equivalent is to multiply the numerator and denominator of each of them by the same number. This process is known as amplification .
Returning to the previous example, we can try number 3 :
(5 x 3) / (10 x 3) = 15 / 30 = 0.5
(15 x 3) / (30 x 3) = 45 / 90 = 0.5
(20 x 3) / (40 x 3) = 60 / 120 = 0.5
Simplification is a similar process, although based on dividing the numerator and denominator by the same number. It is important to note that in order to complete this operation the two terms must be divisible by the number in question. If the result is equal, then we are dealing with equivalent fractions. We can do the test with the previous examples and number 5 :
(5 / 5) / (10 / 5) = 1 / 2 = 0.5
(15 / 5) / (30 / 5) = 3 / 6 = 0.5
(20 / 5) / (40 / 5) = 4 / 8 = 0.5
The usefulness of equivalent fractions lies in the possibility of finding a smaller version of another, which makes a certain calculation less complicated, for example. On the other hand, recognizing two or more equivalent fractions in an operation can simplify it if it allows us to eliminate or associate them.
