
Elliptical is that linked to an ellipse.
The term elliptical comes from the Greek concept elleiptikós and refers to that which is linked to an ellipse . An ellipse, on the other hand, is the area of points on a plane whose sum of distances to two other fixed points is constant.
It can be said that an ellipse is a simple, closed curve that has a pair of axes of symmetry . That which has this shape or a similar one is classified as elliptical.
elliptical movement
In this context we can talk about elliptical movement , a concept of great importance for us since it is applied to that made by the planets around the Sun. It is a limited movement, that is, the object that moves is subjected to an entity higher in the hierarchy, which in this case is the central point around which the trajectory is drawn in the form of an ellipsis.
There is more than one physical system in which elliptical movement can be appreciated: in addition to the movement of the planets, related to the law of universal gravitation proposed by Isaac Newton , we can also mention the one that takes place in a harmonic potential field. In both cases, we are faced with central fields (also known as central force ), because the potential energy of the body that makes the trajectory depends only on the distance at which it is from a point that does not move, the source of the field. (also called center ).
If we rely on the Newtonian theory of gravitation, two of the concepts that we must take into account to study the elliptical movement of a particle in a gravitational field whose symmetry is spherical will be the following: the eccentricity of the orbit, that is how much it deviates from what would be a perfect circumference; the angular momentum per unit of mass, that is, how much the object rotates for each unit of mass.
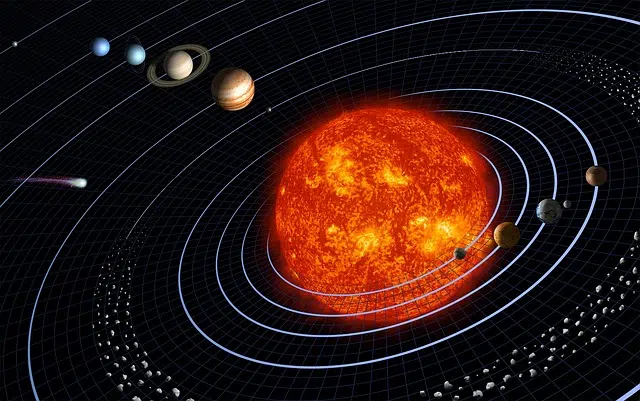
The planets that orbit the Sun perform an elliptical motion.
The harmonic potential
With respect to the harmonic potential , we can think of a ball attached by means of an elastic tape to a point whose position remains unchanged. If the ball begins to move, given the characteristics of this system, its movement will be elliptical and will be composed of two movements whose directions will be perpendicular to each other.
The amplitude of these perpendicular movements will also be different: the highest value will correspond to the semi-minor axis of the ellipse, while the lowest, with the semi-major axis. Furthermore, it is important to mention that there will be a phase shift between them due to angular momentum.
The elliptical in language
The notion of elliptical, meanwhile, is also used to refer to what is associated with an ellipsis . The idea of ellipsis appears in literary theory, rhetoric, and grammar.
The omission of an element, a segment or a sequence in a speech is called an ellipsis. What was omitted with the ellipsis can be recovered thanks to the context, with which the receiver is in a position to reconstruct the meaning. This does not mean that the words left out by the ellipsis affect the grammatical correctness of the sentence.
The action of dispensing with a linguistic element is called eliding . What is elided can be described as elliptical.
Ellipses appear frequently in everyday language. Suppose a child wants to tell a friend what his brothers do. "My sister plays soccer and my brother plays tennis," says the little boy. As you can see, "play" becomes an elliptical component because the verb is omitted in the second part of the statement. Therefore, without ellipsis, the expression would develop as follows: "My sister plays soccer and my brother plays tennis."
