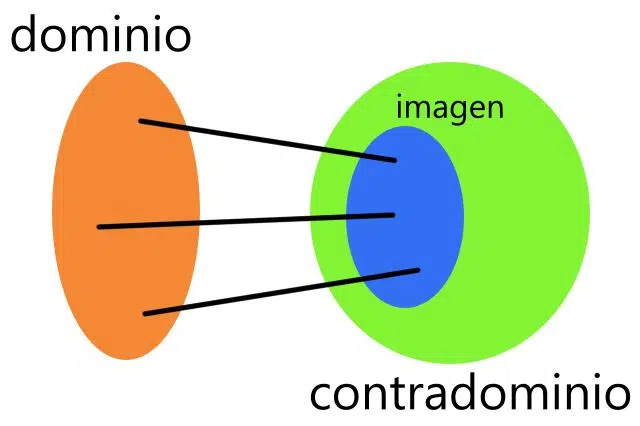
The counterdomain is the arrival set, and includes the image.
The idea of contradomain , also mentioned as arrival set , final set or co-domain , is used in the field of mathematics . The concept appears when referring to functions .
mathematical function
Before moving forward, it is important to remember that a mathematical function is the relationship established between two sets, through which each element found in the first set is assigned a single element (or none) of the second set.
This concept is of great importance in the field of mathematics, but also beyond its limits, since in our daily lives there are endless relationships that can be analyzed as functions . Whether we are aware of it or not, we establish many logical links between the elements that make up our lives, just as we apply the fundamentals of physics to move and avoid obstacles when walking.
Although in the student stage we are accustomed to studying the functions as part of exercises previously thought out by the teachers, so that there is already a solution detected by them, in practice they are proposed to find said solution, so that we know its components ( domain, counterdomain, image, variables, sets of numbers, etc.) and learning to use them is essential.
Domain and counterdomain
The starting set is the domain ; the arrival set , the counterdomain . Therefore, if we consider a set A and a set B , a function is the link that is generated when each element of A (the domain) is assigned a single element of B (the counterdomain).
Let's take the case of the function f(x) = 3. x , with a domain that, in this case, is the set {2, 4, 8} . Therefore, the counterdomain could be the set {6, 12, 24} , since the function consists of multiplying each element of the domain by three.
It is important to keep in mind that the function is a law that presents a one-to-one correspondence . This means that each element of the domain can only correspond to one element of the counterdomain.
In colloquial language , the domain is the set that indicates what can enter the function, while the counterdomain is formed with what is possible to exit. What actually comes out of the function is called an image or range . It should be noted that all functions have their domain.
Difference with the picture
Although it is possible that the terms image and counterdomain may be confused in some context, their technical definition distinguishes them perfectly: the former only has elements that are output values , while the counterdomain may have others, beyond those that correspond. with those of the domain.
The image rarely matches the counterdomain.
Let's look at an example to better understand this difference: if the function consists of multiplying the variable x by 2, then we can safely state that the image only contains even numbers; This is because there is no odd number that is the product of a multiplication by 2 of a natural number (to obtain 3, for example, we must multiply 1.5 by 2, just as 27 is the result of 13.5 by 2 , and so on). The contradomain, on the other hand, is the set of natural numbers, since among them we find the pairs just mentioned.
On the other hand, this difference does not prevent the fact that, in some cases, there is a total coincidence between the image and the counterdomain. In this case, we would find ourselves facing a surjective, suprajective, epijective, subjective, exhaustive or surjective function . In other words, this type of function is characterized by having for each element of the arrival set at least one output. An example of the many possible surjective functions is the following: f(x) = 2 (x + 1) .
