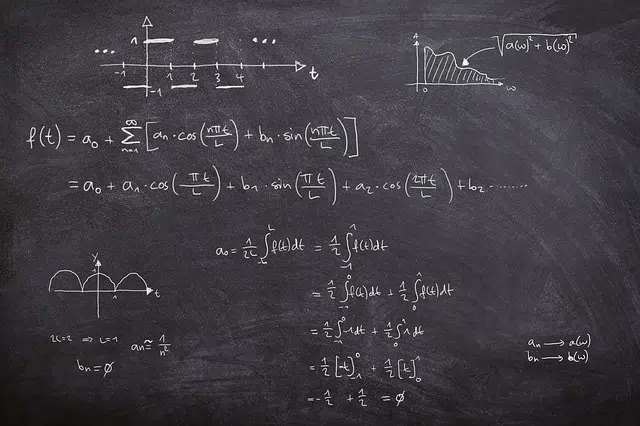
The antiderivative is a kind of mathematical function.
An antiderivative is a mathematical function that is obtained from the opposite process of differentiation . To understand what the notion refers to, therefore, we must first be clear about what a function is in the field of mathematics and what the derivation consists of.
Function and derivative
A function is a relationship established between two sets, which assigns to each of the elements of the first set an element that is part of the second set or none. The group formed by all mathematical entities that have the same property is called a set .
The derivative of a function , on the other hand, is the rate of change with which the value of the function is modified based on an alteration of the value of its independent variable. The derivation indicates the rate at which the function changes based on changes in the independent variable.
Concept of antiderivative
Let us now return to the idea of antiderivative. The function F(x) +C is called the antiderivative of a function f(x) , where C is constituted as a constant .
Thus, by differentiating F(x)+C , we obtain f(x) . That is why the function F(x) is antiderivative of the function f(x) .
In simpler words, we can say that it is the inverse relationship that exists in a derivative. If we take a very simple example, the expression x 2 (x raised to the square or the second power), we know that its derivative is 2 x (2 raised to the power of x). Now, to obtain the antiderivative we have to go the opposite way: the antiderivative of 2 x is, in effect, x 2 .
The integration process
The process carried out to discover antiderivatives (also known as primitives ) is called integration . On the other hand, indefinite integrals make up the family of functions obtained through this process.
It should be noted that, when a function f allows an antiderivative over an interval , it admits an infinity with a constant difference between them.
Continuous function
This concept is fundamental to understand the antiderivative, since for a given function to admit an antiderivative on an interval it is enough for it to be of continuous type. A continuous function, therefore, is one in which a continuous variation in its argument leads to a continuous variation in the value of the function.
Here we are faced with a new concept that needs to be explained to understand the definition: the argument of a function . It is a value that is provided to obtain its result. Another name by which it is known is independent variable , which is mentioned in a previous paragraph. When we talk about a "continuous variation" we mean a change without abrupt jumps (or discontinuity ) in value.
In other words, a function is considered continuous if making small changes to its argument is enough to obtain small arbitrary changes in its value.
There are different types of mathematical functions.
Properties of the antiderivative
Let's start with linearity : the antiderivative is linear. This means that if a function f admits an antiderivative F over an interval I , for any real number k , an antiderivative of kf over the same interval has to be kF .
Another property of the antiderivative is that if we apply it to an odd function, the result is always even. We can also affirm that to obtain the antiderivative of a periodic function (one whose values are repeated at regular intervals) we must add a linear function (a function that has a single variable and that in the Cartesian plane is represented as a straight line) and a periodic .
