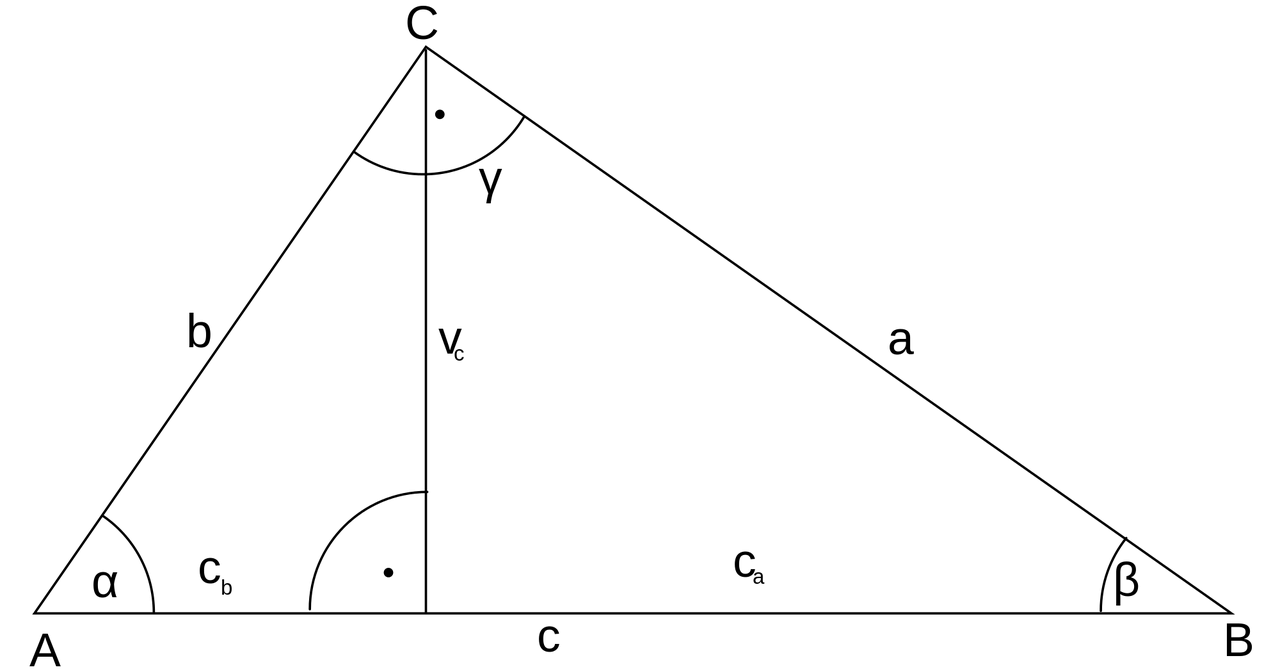
An angle is formed when two lines intersect each other.
Angle is a notion that comes from the Latin word angŭlus and refers to a geometric figure that is formed from two straight lines that intersect each other on the same surface. It can also be said that an angle is formed by two rays that share the same vertex.
Angles can be measured in different units : the sexagesimal degree and the radian are the most common measurements. According to this measurement, angles are classified in different ways.
If we place ourselves in the field of sexagesimal degrees, a right angle , for example, measures 90°. If it measures less than 90° but more than 0°, it is referred to as an acute angle . On the other hand, if it measures more than 90° and less than 180°, it is called an obtuse angle .
Measurement of an angle
The unit that is first taught in school is the sexageximal degree , since it is easier to understand: with the help of a measuring instrument , such as the protractor, we must determine the opening of the angle and assign the corresponding value, in a similar way to what we do when measuring the extension of an object in centimeters. However, the radian is much more useful and is predominantly used in the scientific environment.
To carry out the measurement of an angle in radians we must continue its arc until completing an imaginary circle , in the center of which the vertex of the first is located; In other words, we can think of a cake that is missing a slice, this being the angle to measure. The value of 1 radian is the equivalent of the arc whose length is, in turn, equal to the radius of the circle in question; Half the circle is π (pi) radians, while 2π radians is the full circle . Converting a value in sexagesimal degrees to radians consists of multiplying it by pi and dividing it by 180.

A quadrilateral has four interior angles.
Trigonometry
Trigonometry is the branch of mathematics that specializes in measuring triangles. Specifically, what it does is study trigonometric ratios , such as the tangent , the sine , the secant , the cotangent , the cosine and the cosecant .
These operations that revolve around angles are key in GPS and astronomy , for example, since through triangulation techniques it is possible to measure the distances between stars or between geographical locations.
A bisector allows an angle to be divided into two angles of identical measure.
Classification of angles according to type
Null angle , straight angle , concave angle , and complete angle are some of the most common types.
Also, taking other characteristics , we can speak of adjacent angle , supplementary angle , complementary angle , exterior angle , interior angle , reflection angle , conjugate angle or solid angle , among others.
Its importance in the development of digital graphics
In the field of computer graphics development, which encompasses various forms of modern entertainment such as cinema and video games, the concept of angle is among the most relevant, as it appears in various situations: the point of view of the camera, the direction in which an object moves, the rotation of different parts of an animated model, collisions between two objects (such as the ground and a character or two characters) and the influence of wind on the stage are as just some examples.
Unlike other operations , such as addition and multiplication, the calculation necessary to find the value of an angle is relatively demanding for a processor, as is that of the square root, and that is why programmers must find "economical" methods to do so. avoid runtime overhead; A very common solution is to calculate all the necessary values during program loading, to create a list that can later be consulted without problem.
The concept of angle in colloquial language
Beyond the limits of geometry, the idea of angle is often used to name a corner or corner : "I think we could place the new library in that corner," "Grandma's vase shines in a corner of the dining room." » .
Angle, on the other hand, is a perspective or a point of view . It is said that a person observes reality according to his or her own particular view, known as an angle: "From my angle, experience is the most important thing to successfully carry out this type of task."
Suppose that two sports journalists develop an analysis of a soccer match that ended in a tie. For one of the analysts, the result favored the local team since it allowed them to remain three points behind the championship leader, while their rival accumulated their fourth game without winning. The other reporter, on the other hand, maintains that the draw benefited the visitor because it helped them break a streak of three consecutive defeats, while their opponent failed to get closer to the top of the standings, as was their objective. As can be seen, both chroniclers analyze the situation from different angles .
